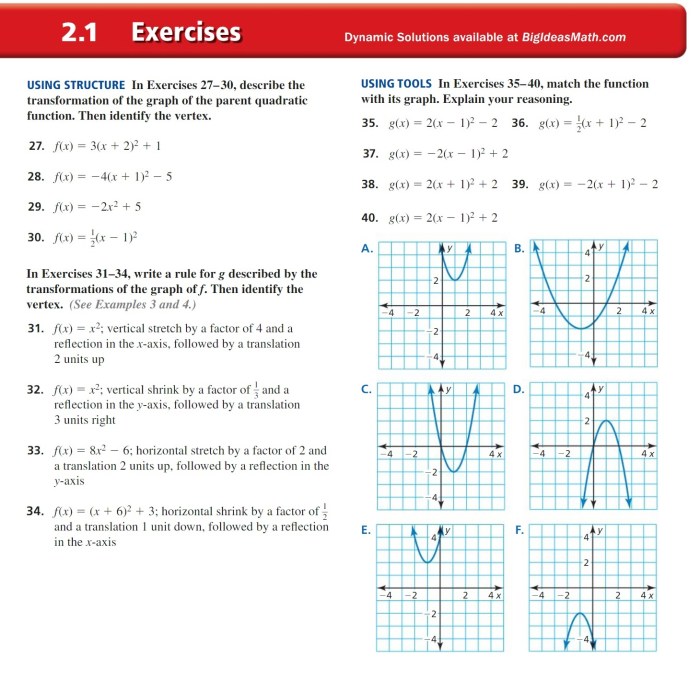
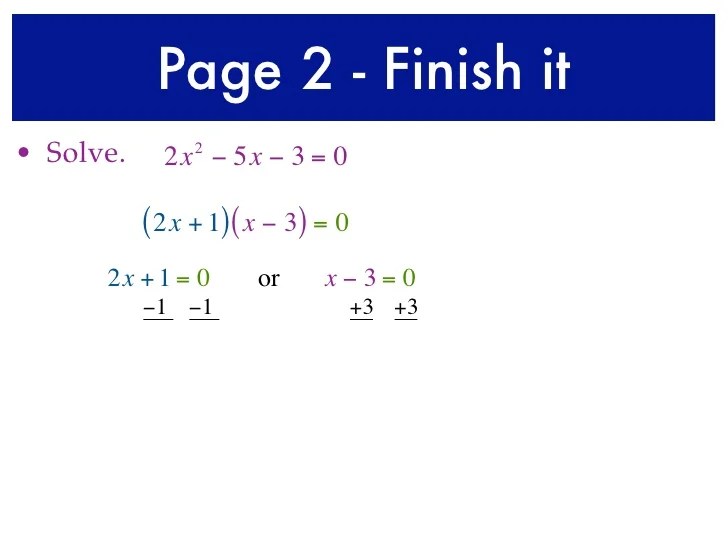
Embarking on a journey through nc math 1 unit 7 building quadratic functions, this comprehensive guide unveils the intricacies of these essential mathematical concepts. From their foundational principles to their practical applications, we delve into the world of quadratic functions, exploring their unique characteristics, graphical representations, and problem-solving capabilities.
Through engaging explanations, illustrative examples, and real-world scenarios, we unravel the complexities of quadratic functions, empowering learners with the knowledge and skills to navigate this fascinating mathematical landscape.
Building Quadratic Functions

Quadratic functions are a type of polynomial function that has a degree of 2. They are defined by the general equation y = ax^2 + bx + c, where a, b, and c are real numbers and a is not equal to 0. Quadratic functions are commonly used to model a variety of real-world phenomena, such as the trajectory of a projectile, the growth of a population, and the shape of a parabola.
To build a quadratic function, you need to know the values of the coefficients a, b, and c. The coefficient a determines the shape of the parabola, the coefficient b determines the position of the vertex, and the coefficient c determines the y-intercept.
Once you know the values of the coefficients, you can plug them into the general equation to get the equation of the quadratic function.
Graphing Quadratic Functions, Nc math 1 unit 7 building quadratic functions
To graph a quadratic function, you can use the following steps:
- Find the vertex of the parabola. The vertex is the point where the parabola changes direction. The x-coordinate of the vertex is
b/2a, and the y-coordinate of the vertex is f(-b/2a).
- Find the y-intercept. The y-intercept is the point where the parabola crosses the y-axis. The y-intercept is (0, c).
- Find the x-intercepts. The x-intercepts are the points where the parabola crosses the x-axis. To find the x-intercepts, set y = 0 and solve for x.
- Plot the points you found in steps 1-3 and connect them with a smooth curve.
The graph of a quadratic function is a parabola. The parabola opens up if a is positive and opens down if a is negative. The vertex of the parabola is the highest point on the graph if a is positive and the lowest point on the graph if a is negative.
Applications of Quadratic Functions
Quadratic functions are used in a variety of applications, including:
- Modeling the trajectory of a projectile
- Modeling the growth of a population
- Modeling the shape of a parabola
- Solving problems in physics and engineering
Quadratic functions are a powerful tool for modeling a variety of real-world phenomena. They are relatively easy to understand and use, and they can be used to solve a wide range of problems.
Solving Quadratic Equations
Quadratic equations are equations that can be written in the form ax^2 + bx + c = 0, where a, b, and c are real numbers and a is not equal to 0. There are three methods for solving quadratic equations:
- Factoring
- Completing the square
- Quadratic formula
The factoring method is the easiest method to solve quadratic equations, but it only works if the quadratic equation can be factored. The completing the square method is a more general method that can be used to solve any quadratic equation.
The quadratic formula is a general formula that can be used to solve any quadratic equation, but it is usually the least efficient method.
Questions and Answers: Nc Math 1 Unit 7 Building Quadratic Functions
What are quadratic functions?
Quadratic functions are polynomial functions of degree 2, characterized by their distinctive parabolic shape. They are expressed in the general form of f(x) = ax² + bx + c, where a, b, and c are real numbers and a ≠ 0.
How do you graph a quadratic function?
To graph a quadratic function, you can use the following steps: find the vertex, axis of symmetry, and intercepts; plot these points; and sketch the parabola through the points.
What are some applications of quadratic functions?
Quadratic functions have numerous applications in various fields, including physics, engineering, and economics. They can be used to model projectile motion, optimize trajectories, and analyze financial data, among other applications.